Mathematics is the Language in Which God Wrote the Universe
- VKL

- Aug 3, 2024
- 6 min read
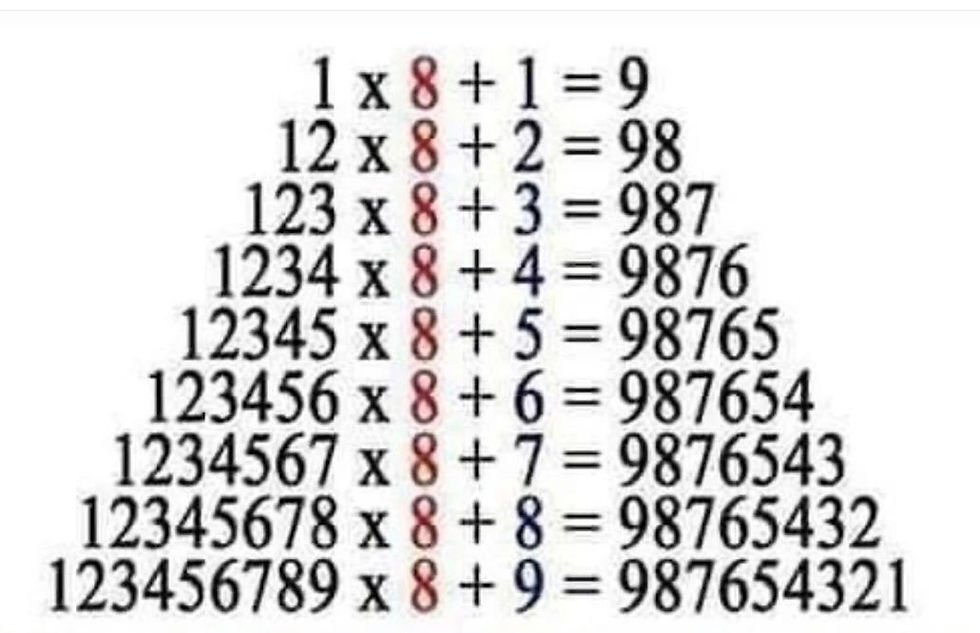
In the grand tapestry of existence, mathematics stands as the thread that weaves together the fabric of reality. Galileo Galilei once famously stated, "Mathematics is the language in which God wrote the universe." This profound assertion encapsulates the essence of mathematics as not just a tool for understanding the world, but as the very language through which the cosmos speaks.
As we delve deeper into the intricate relationship between mathematics and the universe, we uncover the undeniable truth that numbers, equations, and geometric shapes form the foundation of everything we perceive.
The Divine Precision of Mathematical Constants
At the heart of mathematics lies a set of constants that define the universe's behavior with astonishing precision. Pi (π), for instance, is a mathematical constant that represents the ratio of a circle's circumference to its diameter. This seemingly simple number, approximately equal to 3.14159, permeates various aspects of our world, from the orbits of planets to the design of bridges. Its ubiquity underscores the inherent mathematical order in nature.
Similarly, the golden ratio (φ), approximately 1.618, manifests in the patterns of shells, hurricanes, and galaxies. This ratio, often referred to as the "divine proportion," has been employed by artists and architects throughout history to create aesthetically pleasing compositions. The presence of such constants in both natural and human-made structures hints at a universal blueprint governed by mathematical principles.

Geometry: The Architecture of the Universe
Geometry, the branch of mathematics concerned with the properties and relations of points, lines, surfaces, and solids, serves as the architectural framework of the universe.
Euclidean geometry, named after the ancient Greek mathematician Euclid, forms the basis of our understanding of space and shapes. Through Euclidean principles, we comprehend the properties of parallel lines, the angles of triangles, and the areas of polygons.
In contrast, non-Euclidean geometries, such as those developed by Gauss, Lobachevsky, and Riemann, challenge our perceptions of space by exploring curved surfaces and higher dimensions. These geometries are pivotal in the field of general relativity, where Einstein's equations describe the curvature of spacetime around massive objects. The intricate dance of planets, stars, and galaxies follows the geometric patterns dictated by these mathematical laws.

Algebra: The Symbolic Language of Patterns
Algebra, the branch of mathematics dealing with symbols and the rules for manipulating those symbols, provides a powerful language for expressing patterns and relationships. The fundamental operations of addition, subtraction, multiplication, and division, when combined with variables, form the basis of algebraic equations that model real-world phenomena.
Quadratic equations, for example, describe the parabolic trajectories of projectiles, while
exponential functions capture the growth of populations and the decay of radioactive substances. By solving these equations, we unlock the secrets of the natural world, predicting outcomes and optimizing processes. Algebra's versatility extends to the realm of computer science, where algorithms and data structures rely heavily on algebraic concepts to perform complex computations.
Calculus: The Mathematics of Change
Calculus, the study of change and motion, revolutionized our understanding of the universe by providing the tools to analyze dynamic systems. Developed independently by Newton and Leibniz in the 17th century, calculus encompasses two main branches: differential calculus and integral calculus.
Differential calculus focuses on rates of change, allowing us to determine the velocity of a moving object or the slope of a curve at any given point. This branch is instrumental in physics, where it underpins the laws of motion and the principles of thermodynamics.
Integral calculus, on the other hand, deals with the accumulation of quantities, such as the area under a curve or the total distance traveled by an object. It plays a crucial role in engineering, economics, and biology, enabling us to calculate volumes, optimize resources, and understand the behavior of complex systems.
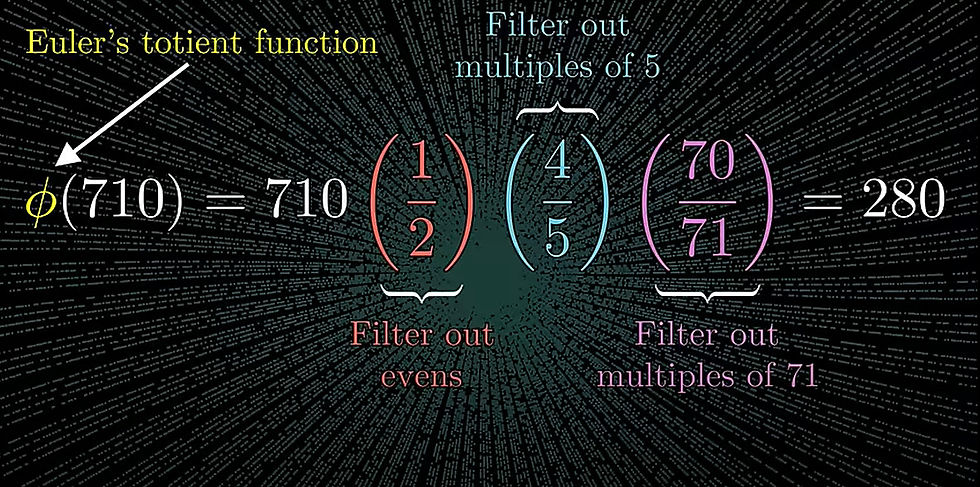
Number Theory: The Mystique of Prime Numbers
Number theory, the study of integers and their properties, delves into the fundamental nature of numbers themselves. Prime numbers, which are divisible only by 1 and themselves, have fascinated mathematicians for centuries. Their distribution appears random, yet they hold the key to some of the most profound mysteries in mathematics.
The Riemann Hypothesis, one of the most famous unsolved problems in mathematics, posits a deep connection between the distribution of prime numbers and the zeros of the Riemann zeta function. Solving this hypothesis would unravel new insights into the structure of numbers and their role in the universe.
Prime numbers also play a critical role in modern cryptography, securing digital communication by providing the foundation for encryption algorithms. The seemingly random nature of primes ensures the security of our online transactions and confidential information.
The Role of Mathematics in Scientific Discovery
Throughout history, mathematics has been the driving force behind scientific discovery. Isaac Newton's formulation of the laws of motion and universal gravitation relied heavily on mathematical principles. His invention of calculus provided the framework for describing the motion of celestial bodies and the forces acting upon them.
In the 20th century, Albert Einstein's theory of general relativity revolutionized our understanding of gravity, space, and time. The mathematical equations he developed to describe the curvature of spacetime have been confirmed by numerous experiments and observations, from the bending of light around massive objects to the detection of gravitational waves.
Quantum mechanics, the branch of physics that describes the behavior of particles at the atomic and subatomic levels, is deeply rooted in mathematical formalism. The probabilistic nature of quantum phenomena is captured through complex wave functions and operators, enabling scientists to predict the outcomes of experiments with remarkable accuracy.
Mathematics in Everyday Life
While the lofty heights of theoretical physics and cosmology may seem distant from our daily lives, mathematics is ever-present in our routines. From calculating the optimal route to work using graph theory to managing personal finances with basic arithmetic, we rely on mathematical concepts to navigate the complexities of modern life.
Statistics and probability help us make informed decisions in the face of uncertainty, whether we are evaluating medical treatments, assessing risks, or interpreting data. The rise of machine learning and artificial intelligence has further amplified the role of mathematics, as algorithms analyze vast datasets to uncover patterns and make predictions.
The Philosophical Implications of Mathematics
Beyond its practical applications, mathematics carries profound philosophical implications. The question of whether mathematical truths are discovered or invented has sparked debates among philosophers and mathematicians alike. Platonists argue that mathematical objects exist in an abstract realm, independent of human thought, while formalists view mathematics as a creation of the human mind, governed by logical rules and symbols.
The uncanny effectiveness of mathematics in describing the physical world, as highlighted by physicist Eugene Wigner, raises intriguing questions about the nature of reality. Why does a language constructed by humans so accurately reflect the workings of the cosmos? This "unreasonable effectiveness" suggests a deep, perhaps inherent, connection between mathematics and the universe.
Conclusion
In the grand symphony of existence, mathematics serves as both the score and the conductor, orchestrating the harmonious interplay of forces and phenomena. From the smallest particles to the vast expanse of the cosmos, mathematical principles underpin the structure and behavior of the universe. As we continue to explore and unravel the mysteries of mathematics, we draw closer to understanding the divine language in which the universe is written.
If you want to read more information about how to boost traffic on your Website just visit --> The Insider's Views
FAQs
1. What are some examples of mathematical constants in nature? Mathematical constants like Pi (π) and the golden ratio (φ) appear in various natural phenomena, including the orbits of planets, the patterns of shells, and the structure of galaxies.
2. How does geometry relate to the physical universe? Geometry, particularly Euclidean and non-Euclidean geometries, provides the framework for understanding the properties of space, shapes, and the curvature of spacetime, as seen in general relativity.
3. Why is algebra important in real-world applications? Algebra allows us to model and solve real-world problems by expressing patterns and relationships through equations, which are essential in fields like physics, engineering, and computer science.
4. What is the significance of calculus in scientific discovery? Calculus, with its focus on change and accumulation, is crucial for analyzing dynamic systems, describing the laws of motion, and understanding complex processes in physics, engineering, and economics.
5. How does number theory contribute to modern technology? Number theory, particularly the study of prime numbers, underpins modern cryptography, ensuring the security of digital communications and safeguarding sensitive information.




Comments